Back to Blog

Read Article

Discussion –

Read Article

Discussion –

STPM Coursework

STPM 2018 Term 3 Mathematics (T) Coursework Sample Answer

By KK LEE

August 12, 2018

STPM 2018 term 3 mathematics (T) Coursework sample

Guideline for solving STPM 2018 Term 3 Mathematics (T) PBS coursework assignment

methodology

Hint: Binomial Theorem

Chi-Squared tests

tabulate

Thanks for helping in doing the survey.

You can find the sample of the results below. Scroll down for all the sample answers and solutions.

Question

A researcher claims that 40% of Malaysians wear glasses. The number of people wearing glasses in a family may be modelled by a binomial distribution. In this assignment, you are required to conduct a study on the number of family members wearing glasses in k-member families with k>4. 1 Comment on the suitability of the binomial model in this situation. 2 By choosing one value of k, take a random sample of at least 50 families with k members. Tabulate the distribution of the number of family members wearing glasses. 3 Test the hypothesis that your sample data is from a binomial distribution with parameter p = 0.4. 4 (a) Explain how the test would be modified if the hypothesis to be tested that your sample data is from a binomial distribution with the parameter p unknown. (b) Carry out this test. 5 Comment on your results.

I have conducted survey on the number of family members wearing glasses in 5-member families. The method i used to conduct the survey is using the Google Form. Thanks to the students who helped to fill in the form.

i. Each family member (trial) has two outcomes; wearing glasses and not wearing glasses.
ii. Fixed number of family members. (5 members)
iii. Independent, The probability of family member wearing glasses is assumed to be independent. We neglect the factors like genetic, races and sex
iv. The probability of success, p, is assumed to be constant for each family member.

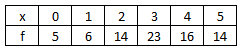
A total of 78 5-members families are chosen. The frequency distributions are tabulated as above.

Chi-squared test is used to test if the sample data is from binomial distribution with parameter p=0.4.

Significance level chosen is 5%.

H0: The number of family members who are wearing spectacles can be modelled by a binomial distribution with parameter p=0.4.

H1: The number of family members who are wearing spectacles cannot be modelled by a binomial distribution with parameter p=0.4.

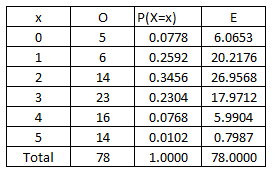
The values of P(X=x) is obtained using the formula of binomial distribution.

The values of E can be obtained by taking the P(X=x) and multiply with 78 (Total families in survey)

The survey was conducted using Google Form and all students are allowed to fill in the form with multiple times (even though they have been warned to fill in once). Therefore the data i used above is taken exactly from the survey from students.

The expected frequency of the category x=5 is less than 5. Therefore the categories x=4 and x=5 are combined.

Please take note that the expected frequencies should not be less than 5.

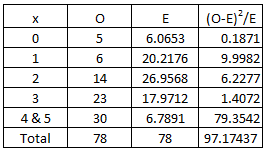
Degree of freedom, v=n-1.

(n=the number of categories, 1=the number of restrictions)

For my case, v=5-1=4.

You can refer to the chi-squared distribution table. For my case, we test using 5% significance level, (we read 0.95 from the table), critical value = 9.488.

We reject H0 if the test statistic is larger than 9.488.

Test statistic = 97.17 (based on the table above).

Since the test statistic 97.17 > 9.488. H0 is rejected and it is enough evidence at 5% significance level, the data does not fit binomial distribution with parameter p=0.4.

Even at 0.1% significance level, H0 is also rejected because the test statistic is large.

(a) When the parameter, p is unknown, we have to estimate the p by using the property of mean of binomial = mean of data.

Another modification will be the degree of freedom. The number of restrictions is 2 now. So degree of freedom = n-2.

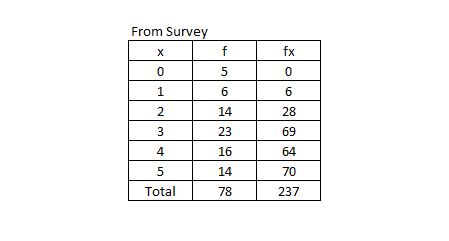
(b) We have to find mean from the data obtained from the survey. Please refer the table below.

Mean = 237/78=3.0384615384615384615384615384615

Mean of binomial = np = 5p

5p = 3.0384615384615384615384615384615

p=0.6076923

H0: The number of family members who are wearing spectacles can be modelled by a binomial distribution with parameter p=0.6076923.

H1: The number of family members who are wearing spectacles cannot be modelled by a binomial distribution with parameter p=0.6076923.

Significance level chosen is 5%.

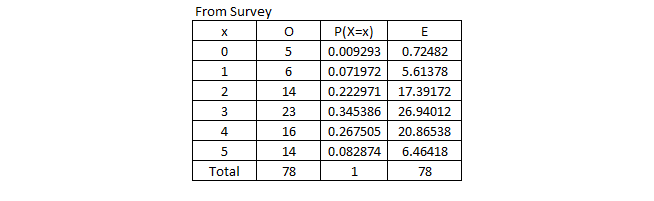
The values of P(X=x) is obtained using the formula of binomial distribution, X ~ B (5, 0.6076923).

The values of E can be obtained by taking the P(X=x) and multiply with 78 (Total families in survey)

The survey data is the same as the Question 3.

The expected frequency of the category x=0 is less than 5. Therefore the categories x=0 and x=1 are combined.

Please take note that the expected frequencies should not be less than 5.

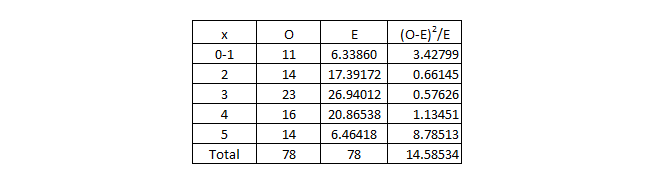
Degree of freedom, v=n-2.

(n=the number of categories, 1=the number of restrictions)

For my case, v=5-2=3.

You can refer to the chi-squared distribution table. For my case, we test using 5% significance level, (we read 0.95 from the table), critical value = 7.815.

We reject H0 if the test statistic is less than 7.815.

Test statistic = 14.58534 (based on the table above).

Since the test statistic 14.58534 > 7.815. H0 is rejected and it is enough evidence at 5% significance level, the data does not fit binomial distribution with parameter p=0.6076923.

But at 0.1% significance level, H0 is not rejected because the test statistic is smaller compare the critical value (16.27).

At any level of significance, the sample data does not fit binomial distribution with p = 0.4.
The Chi-square test show that the sample data does not fit binomial distribution with p = 0.6076923 for any level of significance, except 0.1%.

Here are our most recent updates posts

- Feel free to check it out -

STPM 2015 MT Chapter 8 Booklet A Page 20 Problem 11 Question 14

KK LEE Booklet Solution

Given that $y=\sec^{-1}(e^x)$ , prove that $\frac{dy}{dx}=\frac{1}{\sqrt{e^{2x}-1}}$ .

2015 MT Chapter 8 Booklet A Page 20 Problem 11 Question 11

KK LEE Booklet Solution

Given that $y=(\sin^{-1}x)^2$ , show that $(1-x^2)\frac{d^2y}{dx^2}-x\frac{dy}{dx}-2=$ 0.

STPM 2013 Mathematics (T) and (M) Final Grade

STPM Reference

This is the collection of the final grade of the new STPM Mathematics (T) year 2013. The final grade of Mathematics (T) is based on the grade of Paper 1, Paper 2, Paper 3, and Paper 4(PBS). All the exam marks are hidden and it is hard for STPM students to determine...

2015 MT Chapter 8 Booklet A Page 20 Question 6

KK LEE Booklet Solution

Given that $y=\ln(1+x)-x+\frac{1}{2}x^2$ show that $\frac{dy}{dx}>=0$ for all values of $x>-1$ .

2015 MT Chapter 8 Booklet A Page 20 Question 10

KK LEE Booklet Solution

If $\sin y = 2 \sin x$ , show that (a) $(\frac{dy}{dx})^2=1+3\sec^2 y$ , (b) by differentiating (a) with respect to $x$ , show that $\frac{d^2y}{dx^2}=3\sec^2 y \tan y$ , and hence that $\cot y \frac{d^2y}{dx^2}-(\frac{dy}{dx})^2+1=$ $0$ .

2015 MT Chapter 8 Booklet A Page 20 Question 8

KK LEE Booklet Solution

Given $y=\ln(\sin px + \cos px)$ . Prove that $\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2+p^2=0$ .

Tags: Binomial Distribution Chi-square test MT Term 3 STPM 2018 STPM MT

← Previous Post Next Post →

KK LEE

KK LEE has been a STPM Mathematics tuition teacher since June 2006, but his love of Maths dates back to at least 1999 when he was Form 4. KK LEE started teaching in 2006 at Pusat Tuisyen Kasturi. He was known as "LK" when he was teaching in PTK. After teaching STPM Mathematics for 8 years in PTK, he joined Ai Tuition Centre in 2014. Over the years he has taught Mathematics (T), Mathematics (S), Mathematics (M), Additional Mathematics.

34 Comments

Shining on August 16, 2018 at 2:00 am

this is great help but Q4 and 5 are missing…
Reply
TJY on August 22, 2018 at 2:07 pm

i want to ask that the hypothesis test same as the chi squared test in Q3? Or the chi squared test is a part of the hypothesis test?
Reply
- KK LEE on August 24, 2018 at 11:09 am
  
  Chi-squared test is hypothesis test.
Buddy on August 23, 2018 at 11:45 pm

Thank you so much for the guides. Really helping.
Reply
Yap Gay Chin on August 24, 2018 at 12:35 pm

How do you calculate P(X=x) for Question 3 ? I calculated it by applying binomial distribution formula but I dont seem to be able to obtain the same answer as yours after several attempts.
Reply
- KK LEE on August 24, 2018 at 9:47 pm
  
  5Cx (0.4)^x (0.6)^(5-x)
Yap Gay Chin on August 24, 2018 at 9:41 pm

What is the reason to increase the number of constrictions to 2 for degree of freedom , but not to 3 or 4 ? In question 4 .
Reply
- KK LEE on August 24, 2018 at 9:46 pm
  
  Degree of freedom, v=n-1. (n=the number of categories, 1=the number of restrictions) when parameter is known.
  
  The number of restrictions is 2 when the parameter is unknown. So degree of freedom = n-2.
  
  It is mentioned in the post above. If you need further explanation, please google.
Limlong on August 25, 2018 at 8:18 am

Why for the question to do the hypothesis test for unknown parameter must be using degree of freedom v = n-2？
Reply
- KK LEE on August 26, 2018 at 7:26 pm
  
  Degree of freedom, v=n-1. (n=the number of categories, 1=the number of restrictions) when parameter is known.
  
  The number of restrictions is 2 when the parameter is unknown. So degree of freedom = n-2.
  
  It is mentioned in the post above. If you need further explanation, please google.
Bling bling on August 25, 2018 at 3:59 pm

Is the question 5 answer this short only?
Reply
- KK LEE on August 26, 2018 at 7:23 pm
  
  It depends on your teacher.
Lim Jun Tong on September 2, 2018 at 3:35 pm

Can I do 4 categories combined into one? Because the expected value of the 4 categories from my survey is too small.
Reply
- KK LEE on September 26, 2018 at 9:53 am
  
  You should increase the total n
Hs on September 6, 2018 at 8:08 pm

Is there the first is the answer for the comment of suitability of binomial？
Reply
- KK LEE on September 26, 2018 at 9:53 am
  
  You can explain in more details in your submission
Hs on September 6, 2018 at 8:10 pm

Is that the first one is the answer for comment of the suitability of using binomial？
Reply
Yesung on September 10, 2018 at 6:02 pm

Can u give some tips about to how write the methodology?
Reply
lim xx on September 11, 2018 at 5:21 pm

can you write out the conclusion？
thank you so much！
Reply
Olivia 1932 on September 11, 2018 at 8:54 pm

Thank you for the reference for all of us form 6 students. It helps us a lot. You have my upmost gratitude 🙂
Reply
- KK LEE on September 25, 2018 at 10:35 pm
  
  You are welcome.
Olivia 1932 on September 11, 2018 at 8:55 pm

Thank you for the reference for all of us form 6 students for all terms. It helps us a lot. You have my upmost gratitude 🙂
Reply
- KK LEE on September 25, 2018 at 10:36 pm
  
  You are welcome.
Mulan on September 21, 2018 at 12:33 am

In conclusion parameter p known (0.4) or p unknown is more suitable?
Reply
- KK LEE on September 25, 2018 at 10:36 pm
  
  Both can be suitable. It depends on how you explain it.
Mulan on September 21, 2018 at 12:35 am

Which one is more suitable to conduct this survey? With p known or unknown?
Reply
Mulan on September 21, 2018 at 12:36 am

Which one is more suitable to conduct this survey? With p known or p unknown?
Reply
Mulan on September 21, 2018 at 8:39 am

What is the conclusion? Which 1 is more suitable?
Reply
Joan Chin on September 22, 2018 at 6:31 pm

Thanks a lot for the reference. But I would like to ask about my test statistic. Both of it are very large and when p=0.4 and p is unknown, H null are both rejected too. Then, could I write this statement:”the result show p-cap=0.275 is more suitable than the parameter p=0.4?” in the conclusion?
Reply
- KK LEE on September 25, 2018 at 10:44 pm
  
  Yes
Mulan on September 22, 2018 at 6:37 pm

It’s regarding the conclusion.. My first result with p=0.4,Ho is rejected at 5%sl.. Then with p unknown, Ho is rejected at any level of sl except 0.01%. So my conclusion which one is more suitable?
Reply
Joan Chin on September 22, 2018 at 6:49 pm

May i ask is it suitable for me to write “the result shows that the parameter, p-cap=0.275 is more suitable than the parameter, p=0.4” in the conclusion as both of my test statistics are very large no matter for p=0.4 or when p is unknown, and the H null are both rejected too.
Reply
Shin on September 28, 2018 at 11:42 pm

Is it possible that the test statistics go beyond than 200+???
Reply
shuzhen on October 15, 2018 at 10:31 am

why k must be greater than 4?
Reply