0182016992

kklee@kkleemaths.com

GET STARTED

Tuition Timetable

Check the latest timetable of my tuition classes.

Private Tuition

Contact me at 018-2016992 for more info.

Resources

Complete Resources about past year questions are here.

Ai Tuition

Visit Ai Tuition Website for more subjects tuition.

VIEW ALL SERVICES

Back to Blog

Read Article

Discussion – 

9

Read Article

Discussion – 

9

STPM PBS Assignment

STPM 2016 Mathematics (M) Term 1 Assignment

By KK LEE

August 4, 2015
STPM 2016 MM Term 1 Assignment Coursework answer

Introduction

The binomial theorem is a quick way of expanding a binomial expression that is raised to some power. When the binomial expressions are expanded, is there any type of pattern developing which might help us expand more quickly? It is fun to investigate how it works out in a pattern.

 

Sample Question

Question 1

(a) Expand  (a+ b)^n  for  n=0, 1, 2, \ldots, 6.
(b) What observations can we make about the terms in the expansion of  (a + b)^n?
(c) Write down the general formula for the binomial expansion of  (a + b)^n.
(d) Obtain the general formula for binomial expansion of  (1 + x)^n where n is a rational number, and state the condition for the validity of the binomial expansion with a negative integral exponent or a rational exponent.

Question 2

Deduce the value of

(a)  {n \choose 0}+{n \choose 1}+{n \choose 2}+\ldots+{n \choose n}

(b) {n \choose 0}-{n \choose 1}+{n \choose 2}-\ldots+(-1)^n{n \choose n}

Question 3

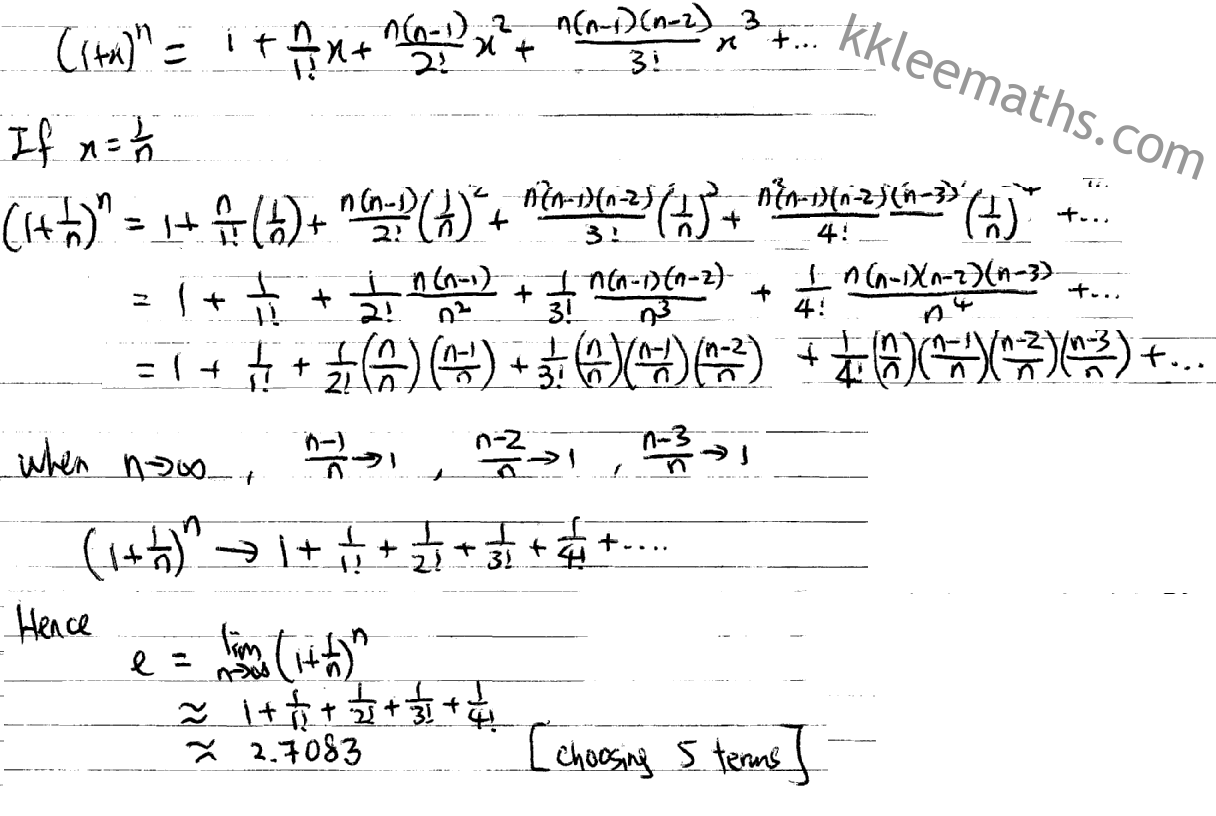
The number e may be defined by e=\lim_{n\to\infty} \left(1+\frac{1}{n}\right)^n. Determine the approximate value for e.

Sample Solution
Scroll down for solution 🙂

Question 1 (a)

Question 1 a Answer

Question 1 (b)

  • There are n+1 terms in the expansion.
  • The first term’s powers start at n and go down.
  • The second term’s powers start at 0 and go up.
  • The coefficients are from Pascal’s triangle or {n \choose r}=\frac{n!}{(n-r)!r!}.

Question 1 (c)

The general term of the expansion  (a+b)^n,  (r+1)-th term is  {n \choose r}a^{n-r} b^r.

Question 1 (d)

In short, (1+x)^n=1+nx+\frac{n(n-1)}{2!}x^2+\frac{n(n-1)(n-2)}{3!}x^3+\ldots+\frac{n(n-1)(n-2)(n-3)\ldots(n-r+1)}{r!}x^r+\ldots. And the expansion is valid for  |x|<1.

Question 2

Question 2 Answer

Question 3

Question 3 Answer

Here are our most recent updates posts

- Feel free to check it out -

STPM Mathematics (T) Term 1 Reference Book

STPM Mathematics (T) Term 1 Reference Book

STPM Term 1 is the toughest term among the three terms in STPM. One of the reasons is the textbook available in the bookstore are not 100% follows the new STPM syllabus. Below are all the reference books that provided in the STPM 2013...

Tags: MM Term 1 Sequence and Series STPM 2016 STPM MM

KK LEE

KK LEE has been a STPM Mathematics tuition teacher since June 2006, but his love of Maths dates back to at least 1999 when he was Form 4. KK LEE started teaching in 2006 at Pusat Tuisyen Kasturi. He was known as "LK" when he was teaching in PTK. After teaching STPM Mathematics for 8 years in PTK, he joined Ai Tuition Centre in 2014. Over the years he has taught Mathematics (T), Mathematics (S), Mathematics (M), Additional Mathematics.

9 Comments

  1. Lai Yee
    Lai Yee on August 5, 2015 at 6:15 pm

    THX…

    Reply
  2. Chia
    Chia on September 13, 2015 at 4:15 pm

    Sir, can u explain question 2

    Reply
    • KK LEE
      KK LEE on September 14, 2015 at 7:54 pm

      To get the equation, you need to substitute x=1 and x=-1

  3. XuanPhua
    XuanPhua on October 11, 2015 at 8:35 pm

    hi, may i know how to write the methodology? i have no idea. QAQ

    Reply
    • KK LEE
      KK LEE on October 13, 2015 at 12:40 am

      For methodology, try to write about the methods/theorem/ways you used to solve the questions.

  4. LKW
    LKW on October 21, 2015 at 11:18 pm

    sir, may i know how to write about “communication”? and what is “viva”

    Reply
    • KK LEE
      KK LEE on October 29, 2015 at 11:17 pm

      It depends on your teacher.

  5. Jh
    Jh on January 12, 2016 at 9:42 pm

    How about term 2 methodology?

    Reply
    • KK LEE
      KK LEE on January 18, 2016 at 12:03 pm

      Hi. Are you referring to Mathematics (M)? I havent receive the question paper. T.T

Submit a Comment

Your email address will not be published. Required fields are marked *

This site uses Akismet to reduce spam. Learn how your comment data is processed.

You May Also Like

No Results Found

The page you requested could not be found. Try refining your search, or use the navigation above to locate the post.